Euronext Paris
Euronext Paris
Indices français
| Indices | Dernier | % |
|---|---|---|
| CAC 40 | 8 091,86 | -0,17 % |
| CAC ALL SHARES | 9 702,88 | -0,20 % |
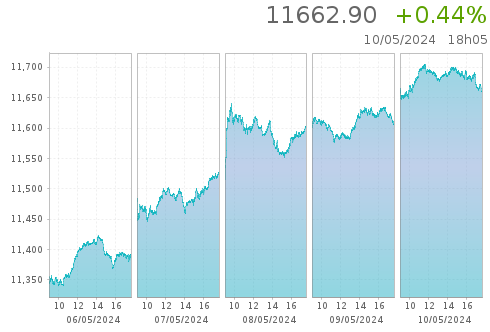
| CAC NEXT 20 | 11 070,56 | -0,47 % |
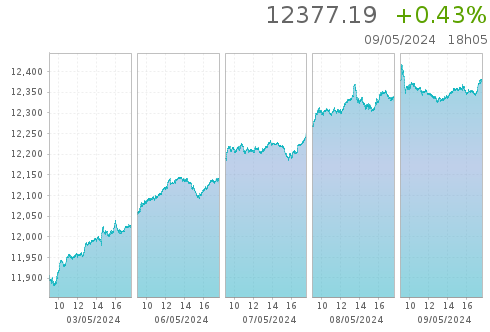
| CAC SMALL | 11 755,08 | -0,10 % |
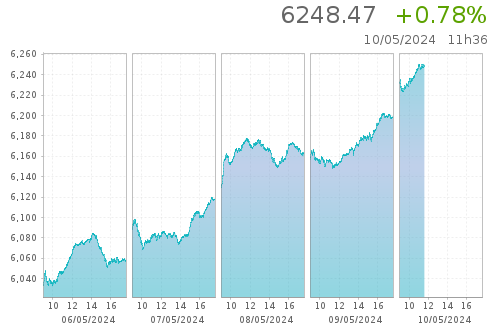
| SBF 120 | 6 112,33 | -0,20 % |
Indices EU
| Instrument-name | Last-price | Day-change-relative |
|---|---|---|
| EURONEXT 100 | 1 515,77 | -0,19 % |
| CLIMATE EUROPE | 1 906,66 | -0,28 % |
| LOW CARBON 100 | 161,61 | -0,47 % |
| NEXT BIOTECH | 2 090,86 | -0,13 % |
| ESG 80 | 2 065,67 | -0,43 % |
Taux de Change
| Instrument-name | Last-price | Day-change-relative |
|---|---|---|
| EUR / USD | 1,07088 | +0,09 % |
| EUR / GBP | 0,85888 | +0,05 % |
| EUR / JPY | 166,6945 | +0,37 % |
| EUR / CHF | 0,9792 | +0,04 % |
| GBP / USD | 1,24684 | +0,05 % |
Indices Francais
CAC 40
CAC ALL SHARES
CAC NEXT 20
CAC SMALL
SBF 120
Marchés au comptant
Marchés dérivés
Communiqués reglementés
Euronext Paris
Pour plus d’information sur Euronext Paris
Visitez la section dédiée sur Euronext.com
